By solving the schrodinger equation (H ̂ = E ̂), we obatin a set of mathematical equation, called wave function ( ̂ ), which describe the probability of finding electrons at certain energy levels within an atom. A wave function for an elctron in an atom calles orbitals; this atomic otbitals describes a region of space in which there is a high probability of finding the electron. Energy changes within an atom are the result of an electron changing from a wave pattern with one energy to wave pattern with different energy ( usually accompained by the absorption or emission of a poton of lights).
Type of orbital’s:
Each orbital is denoted by a number and a letter. The number denotes the energy level of electron in the orbital. This 1 refer to the energy level closest to the nucleus; 2 refer to the next energy level further out, and so on.
The letter refers to the shape of the orbital. The letters go in the orders s, p, d, f, g, h, I, j, etc. The letters s, p, d and f were assigned for historical reasons that need not concern us. All we have to remember the shapes that correspond to each letter.
Since an electron can theoretically occupy all space, it is impossible to draw an orbital. All we can do is draw a shape that will include the electron most of the them, say 95% of the time. We call this shape the 95% contour.
S- Orbital’s:
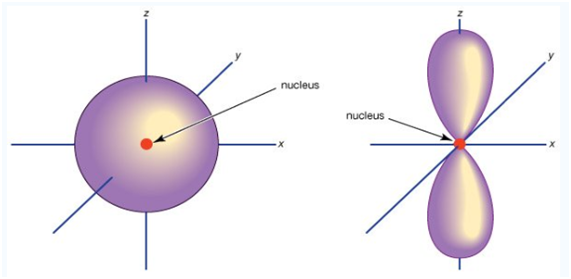
An S orbital is spherically symmetric around the nucleus of the atom, like a hollow ball made of rather fluffy material with the nucleus at its center. As the energy levels increase, the electrons are located further from the nucleus, so the orbitals get bigger. The order of size is 1s ˂ 2s ˂ 3s ˂ …., as shown below:

Now let’s look at a cross –section of these orbitals
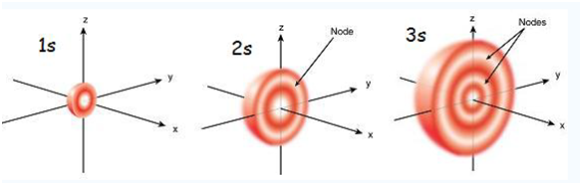
If you look carefully, you will notice that a 1s orbital has very little electron near the nucleus, but its builds up to a maximum as you get further from the nucleus and then decrease the contour. It is sort of like a hollow of like tennis ball.
A 2s orbital is similar to a 1s orbital, but it has sphere of electron density inside the outer sphere, like one tennis ball inside another. There is a surface between the two balls where there is zero probability of finding an electron. We call this surface a node or nodal surface.
A 3s orbital is even larger, and it has three nodes.
p- Orbital’s:
Not all electrons inhabit s orbitals. At the first energy level, the only orbital available to electron is the 1s orbital. However, at the second level, there is also orbital’s called 2p orbital’s in addition to the 2s orbital.
Unlike an s orbital, a p orbital points in a particular direction. The one show below points up and down.
At any one energy level, we have three absolutely equivalent p orbital’s pointing mutually at right angles to each other. These are arbitrarily given the symbols px, py, and pz. This is simply for convenience, because what you might think of as the x, y or z direction changes constantly as the atoms tumbles in space.
d- Orbital’s:
In addition to s and p orbital’s, there are two others set if orbital’s which become available for electrons to inhabit at higher energy levels. At the 3rd level, there is a set of five d orbital’s (with complicated shapes and names) as well as the 3s and 3p orbital’s (3px, 3py, 3pz). At the 3rd level there are a total nine orbital’s altogether.
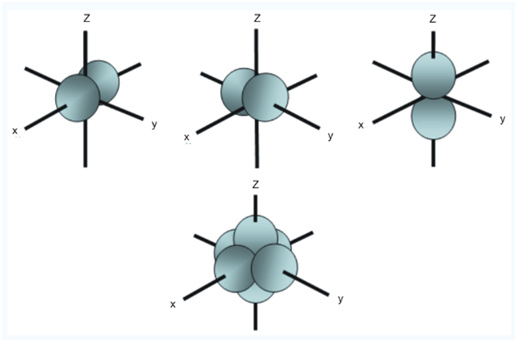
The five 3d orbital’s are under as follow:
- 3dxy
- 3dxz
- 3dyz
- 3dx²
- 3dx²
- 3dz
To make sense of the names, we need to look at them in two groups. The 1st group contains the 3dxy, 3dxz, and 3dyz orbitals. The name tell you that these orbital’s lie in the x-y plane, the x-z plane, and the y-z plane, respectively. Each orbital’s has four lobes, and each of the lobes is pointing between two of the axes, not along them.
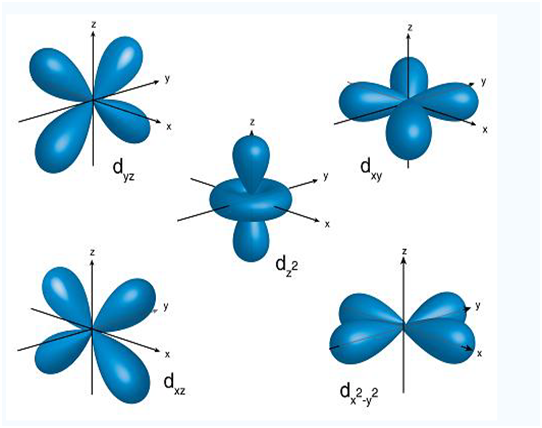
The second group contains the 3dx²-y² and 3dz² orbital’s. Their lobes points along the various axes.
The 3dx²-y² orbital looks exactly like the first group, except that the lobes are pointing along x and y axes, not between them. The 3dz² looks like a p orbital wearing a doughnut around his waist.
f- Orbital’s:,
At the 4th and higher levels, there are seven f orbitals in addition to the 4s, 4p and 4d orbitals.

Counting the 4s, 4p, and 4d orbital’s, this makes a total of 16 orbital’s in the 4th level. They have even more complicated shapes s, p, d, and f orbitals are available at all higher energy levels as well. Fortunately, you will probably not have to memorize the shapes of the f orbitals. Just remember that there are seven f orbital’s in each level from the 4and onwards.
Energy of orbital’s:
The energy which is essential to take an electron present in that orbital to infinity or the release of energy when an electron from an infinity it is added to that orbital, it is referred to as the energy of orbital’s. Each electron in an atom is described by four different quantum numbers. The first three (n, l, mℓ) specify the particular orbital of interest, and the fourth (ms) specifies how many electrons can occupy that orbital.
1: Principal Quantum number (n): n=1, 2, 3……, 8.
Specifies the energy of an electron and the size of the orbital (the distance from the nucleus of the peak in a radial probability distribution plot). All orbital’s that have the same value of n are said to be in the same shell (level). For a hydrogen atom with n=1, the electron is in its ground state; if the electron is in the n=2 orbital, it is in an excited state. The total number of orbital’s for a given n value is n 2.
2: Angular momentum (Secondary, Azimunthal) quantum Number: ℓ=0.., n-1. Specify the shape of an orbital with a particular principal quantum number. The secondary quantum number the shell into smaller groups of orbital’s called subshells (sublevels). Usually, a letter code is used identify ℓ to avoid confusion with n.
| ℓ | 0 | 1 | 2 | 3 | 4 | 5 | …… |
| Letter | s | p | d | f | g | h | …… |
The subshells with n=2 and ℓ=1 is the 2p subshells; if n=3 and ℓ=0 it is the 3s subshells, and so on. The value of ℓ also has a slight effect of the subshells; the energy of the subshells increase with ℓ(s ˂ p ˂ d˂ f).
3: Magnetic quantum number (ml): – ℓ= – 1…… 0…, + ℓ.
Specifies the orientation in space of an orbital of a given energy (n) and (ℓ). This number divides the subshell into individual orbital’s which hold the electrons; there are (2ℓ + 1) orbital’s in each subshell. Thus the s subshell has only one orbital, p subshell has three orbitals, and so on.
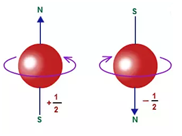
4: Spin quantum number (ms): ms=+½ or -½.
Specifies the orientation of the spin axis of an electron. An electron can spin in only one of two directions (sometime called up and down).